 The Max
von Laue
(1879-1960) equations
relate the incoming waves to the
outgoing waves in the
process of diffraction by a crystal lattice. They can be derived in a
somewhat less formal mode than made by the author,
but intuitively and in a simple way...
The Max
von Laue
(1879-1960) equations
relate the incoming waves to the
outgoing waves in the
process of diffraction by a crystal lattice. They can be derived in a
somewhat less formal mode than made by the author,
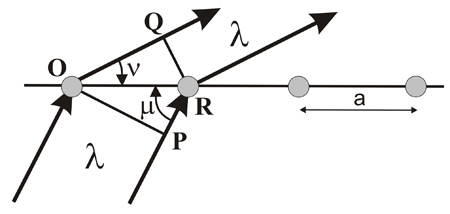
but intuitively and in a simple way...It is enough to consider a row of atoms separated at regular distances, as shown in the figure on the left, and to consider the hypothesis that a wavefront (OP), incident on the line of atoms, scatters cooperatively (diffracts) and produces another wavefront (QR) on the other side of the line whose direction of propagation will have changed.
Therefore, if the wavefront QR is observed (that is, if a cooperative scattering occurs), it is because the two component beams that define that front are in phase. In other words, the difference of paths followed by the beams OQ and PR is an integer number (m) of wavelengths (λ), ie:
OQ
- PR = m λ
and as:cos ν = OQ / a
cos
μ = PR / a
it becomes:a (cos ν - cos μ) =
m λ
If we look carefully at this equation, it is easy to realize that:
Scattering from a row of atoms separated at regular distances
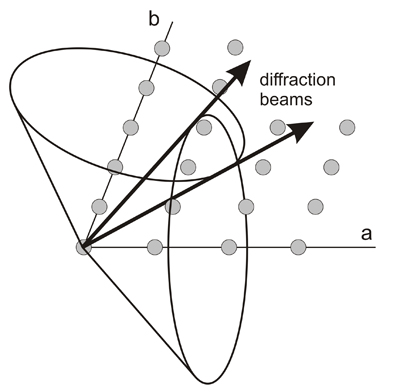
Cooperative scattering (diffraction) from two rows of atoms regularly spaced
In the two-dimensional case, ie if we consider two rows of atoms, such as those shown with the letters a and b (figure above), each one will scatter the X-rays in the same manner as described above, that is in the form of cones coaxial with those atoms rows. But if both dispersions have to be cooperative (diffraction) they will have to perform simultaneously two equations equivalent to the one shown above, ie:
The fact that these two equations are fulfilled simultaneously is equivalent as to consider graphically as valid only the common points of both cones, ie their intersections, which are two straight lines (shown as arrows). In other words, cooperative scattering (diffraction) from two non-parallel rows of atoms (and in general from a plane of atoms) is reduced to discrete diffraction lines only.
Generalizing to any periodically ordered three-dimensional distribution of atoms, we must consider that three equations of the type shown above must be fulfilled simultaneously:
where m, n, p represent three integer numbers. These are the so called Laue equations.
If we look carefully at this equation, it is easy to realize that:
- No fractional cooperative dispersion orders can occur, since m is an integer number, and
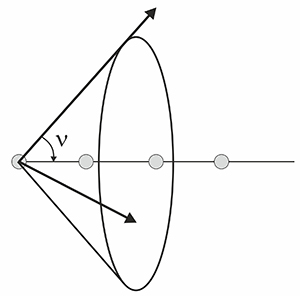
- If the incident angle μ is maintained constant, the last equation will also be valid for any scattered beam that maintains the same angle of scattering (ν) shown in the figure, and therefore all scattered beams around the row of atoms (with the same ν) will fulfill that equation and will form a conical surface coaxial with the row of atoms (see figure below)...

Scattering from a row of atoms separated at regular distances

Cooperative scattering (diffraction) from two rows of atoms regularly spaced
In the two-dimensional case, ie if we consider two rows of atoms, such as those shown with the letters a and b (figure above), each one will scatter the X-rays in the same manner as described above, that is in the form of cones coaxial with those atoms rows. But if both dispersions have to be cooperative (diffraction) they will have to perform simultaneously two equations equivalent to the one shown above, ie:
a (cos ν1 - cos μ1) =
m λ
b (cos ν2 - cos μ2) = n λ
The fact that these two equations are fulfilled simultaneously is equivalent as to consider graphically as valid only the common points of both cones, ie their intersections, which are two straight lines (shown as arrows). In other words, cooperative scattering (diffraction) from two non-parallel rows of atoms (and in general from a plane of atoms) is reduced to discrete diffraction lines only.
Generalizing to any periodically ordered three-dimensional distribution of atoms, we must consider that three equations of the type shown above must be fulfilled simultaneously:
a (cos
ν1 - cos μ1)
=
m λ
b (cos ν2 - cos μ2) = n λ
b (cos ν2 - cos μ2) = n λ
c (cos
ν3 - cos μ3)
= p λ
where m, n, p represent three integer numbers. These are the so called Laue equations.
